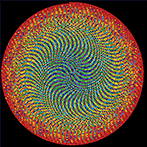
The art of Pi (`\pi`), Phi (`\phi`) and `e`
Numbers are a lot of fun. They can start conversations—the interesting number paradox is a party favourite: every number must be interesting because the first number that wasn't would be very interesting! Of course, in the wrong company they can just as easily end conversations.
The art here is my attempt at transforming famous numbers in mathematics into pretty visual forms, start some of these conversations and awaken emotions for mathematics—other than dislike and confusion

Numerology is bogus, but art based on numbers can be beautiful. Proclus got it right when he said (as quoted by M. Kline in Mathematical Thought from Ancient to Modern Times)
Wherever there is number, there is beauty.
—Proclus Diadochus
 buy artwork
buy artwork
the numbers `\pi`, `\phi` and `e`
The consequence of the interesting number paradox is that all numbers are interesting. But some are more interesting than others—how Orwellian!
All animals are equal, but some animals are more equal than others.
—George Orwell (Animal Farm)
Numbers such as `\pi` (or `\tau` if you're a revolutionary), `\phi`, `e`, `i = \sqrt{-1}`, and `0` have captivated imagination. Chances are at least one of them appears in the next physics equation you come across.
`\pi`
`\phi`
`e`
= 3.14159 26535 89793 23846 26433 83279 50288 41971 69399 ... = 1.61803 39887 49894 84820 45868 34365 63811 77203 09179 ... = 2.71828 18284 59045 23536 02874 71352 66249 77572 47093 ...
Of these three transcendental numbers, `\pi` (3.14159265...) is the most well known. It is the ratio of a circle's circumference to its diameter (`d = \pi r`) and appears in the formula for the area of the circle (`a = \pi r^2`).
 buy artwork
buy artwork
The Golden Ratio (`\phi`, 1.61803398...) is the attractive proportion of values `a > b` that satisfy `{a+b}/2 = a/b`, which solves to `a/b = {1 + \sqrt{5}}/2`.
The last of the three numbers, `e` (2.71828182...) is Euler's number and also known as the base of the natural logarithm. It, too, can be defined geometrically—it is the unique real number, `e`, for which the function `f(x) = e^x` has a tangent of slope 1 at `x=0`. Like `\pi`, `e` appears throughout mathematics. For example, `e` is central in the expression for the normal distribution as well as the definition of entropy. And if you've ever heard of someone talking about log plots ... well, there's `e` again!
Two of these numbers can be seen together in mathematics' most beautiful equation, the Euler identity: `e^{i\pi} = -1`. The tau-oists would argue that this is even prettier: `e^{i\tau} = 1`.
accidentally similar
Did you notice how the 13th digit of all three numbers is the same (9)? This accidental similarity generates its own number—the Accidental Similarity Number (ASN).
Nasa to send our human genome discs to the Moon
We'd like to say a ‘cosmic hello’: mathematics, culture, palaeontology, art and science, and ... human genomes.



Comparing classifier performance with baselines
All animals are equal, but some animals are more equal than others. —George Orwell
This month, we will illustrate the importance of establishing a baseline performance level.
Baselines are typically generated independently for each dataset using very simple models. Their role is to set the minimum level of acceptable performance and help with comparing relative improvements in performance of other models.
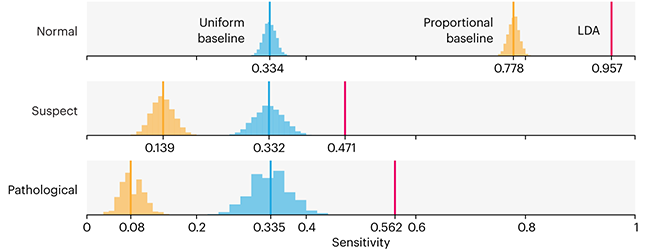
Unfortunately, baselines are often overlooked and, in the presence of a class imbalance5, must be established with care.
Megahed, F.M, Chen, Y-J., Jones-Farmer, A., Rigdon, S.E., Krzywinski, M. & Altman, N. (2024) Points of significance: Comparing classifier performance with baselines. Nat. Methods 20.
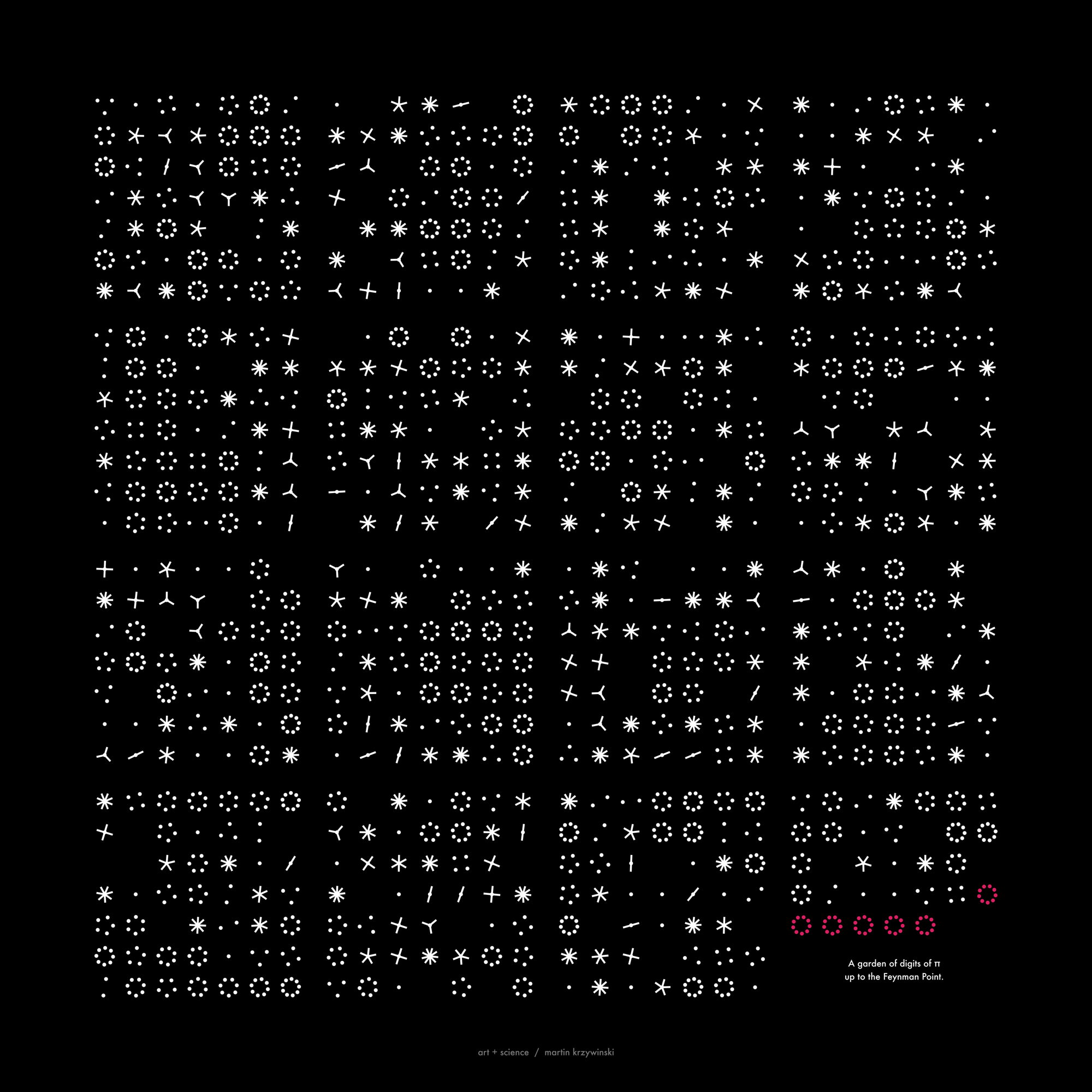
Happy 2024 π Day—
sunflowers ho!
Celebrate π Day (March 14th) and dig into the digit garden. Let's grow something.

How Analyzing Cosmic Nothing Might Explain Everything
Huge empty areas of the universe called voids could help solve the greatest mysteries in the cosmos.
My graphic accompanying How Analyzing Cosmic Nothing Might Explain Everything in the January 2024 issue of Scientific American depicts the entire Universe in a two-page spread — full of nothing.
The graphic uses the latest data from SDSS 12 and is an update to my Superclusters and Voids poster.
Michael Lemonick (editor) explains on the graphic:
“Regions of relatively empty space called cosmic voids are everywhere in the universe, and scientists believe studying their size, shape and spread across the cosmos could help them understand dark matter, dark energy and other big mysteries.
To use voids in this way, astronomers must map these regions in detail—a project that is just beginning.
Shown here are voids discovered by the Sloan Digital Sky Survey (SDSS), along with a selection of 16 previously named voids. Scientists expect voids to be evenly distributed throughout space—the lack of voids in some regions on the globe simply reflects SDSS’s sky coverage.”
voids
Sofia Contarini, Alice Pisani, Nico Hamaus, Federico Marulli Lauro Moscardini & Marco Baldi (2023) Cosmological Constraints from the BOSS DR12 Void Size Function Astrophysical Journal 953:46.
Nico Hamaus, Alice Pisani, Jin-Ah Choi, Guilhem Lavaux, Benjamin D. Wandelt & Jochen Weller (2020) Journal of Cosmology and Astroparticle Physics 2020:023.
Sloan Digital Sky Survey Data Release 12
Alan MacRobert (Sky & Telescope), Paulina Rowicka/Martin Krzywinski (revisions & Microscopium)
Hoffleit & Warren Jr. (1991) The Bright Star Catalog, 5th Revised Edition (Preliminary Version).
H0 = 67.4 km/(Mpc·s), Ωm = 0.315, Ωv = 0.685. Planck collaboration Planck 2018 results. VI. Cosmological parameters (2018).
constellation figures
stars
cosmology
Error in predictor variables
It is the mark of an educated mind to rest satisfied with the degree of precision that the nature of the subject admits and not to seek exactness where only an approximation is possible. —Aristotle
In regression, the predictors are (typically) assumed to have known values that are measured without error.
Practically, however, predictors are often measured with error. This has a profound (but predictable) effect on the estimates of relationships among variables – the so-called “error in variables” problem.
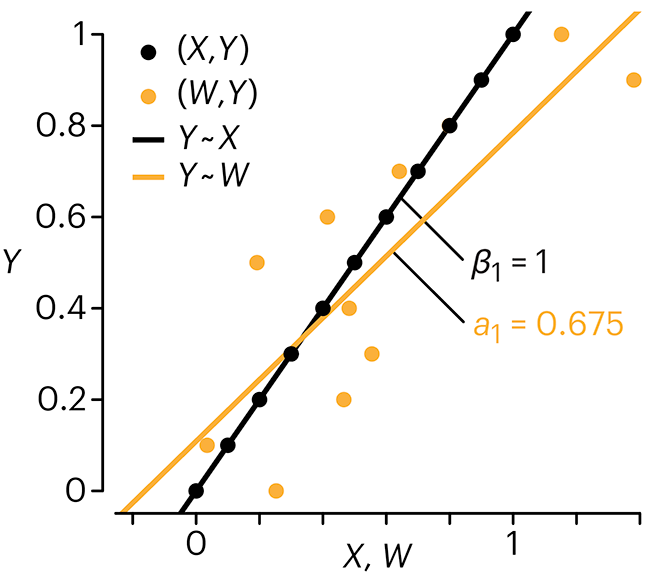
Error in measuring the predictors is often ignored. In this column, we discuss when ignoring this error is harmless and when it can lead to large bias that can leads us to miss important effects.
Altman, N. & Krzywinski, M. (2024) Points of significance: Error in predictor variables. Nat. Methods 20.
Background reading
Altman, N. & Krzywinski, M. (2015) Points of significance: Simple linear regression. Nat. Methods 12:999–1000.
Lever, J., Krzywinski, M. & Altman, N. (2016) Points of significance: Logistic regression. Nat. Methods 13:541–542 (2016).
Das, K., Krzywinski, M. & Altman, N. (2019) Points of significance: Quantile regression. Nat. Methods 16:451–452.
Convolutional neural networks
Nature uses only the longest threads to weave her patterns, so that each small piece of her fabric reveals the organization of the entire tapestry. – Richard Feynman
Following up on our Neural network primer column, this month we explore a different kind of network architecture: a convolutional network.
The convolutional network replaces the hidden layer of a fully connected network (FCN) with one or more filters (a kind of neuron that looks at the input within a narrow window).
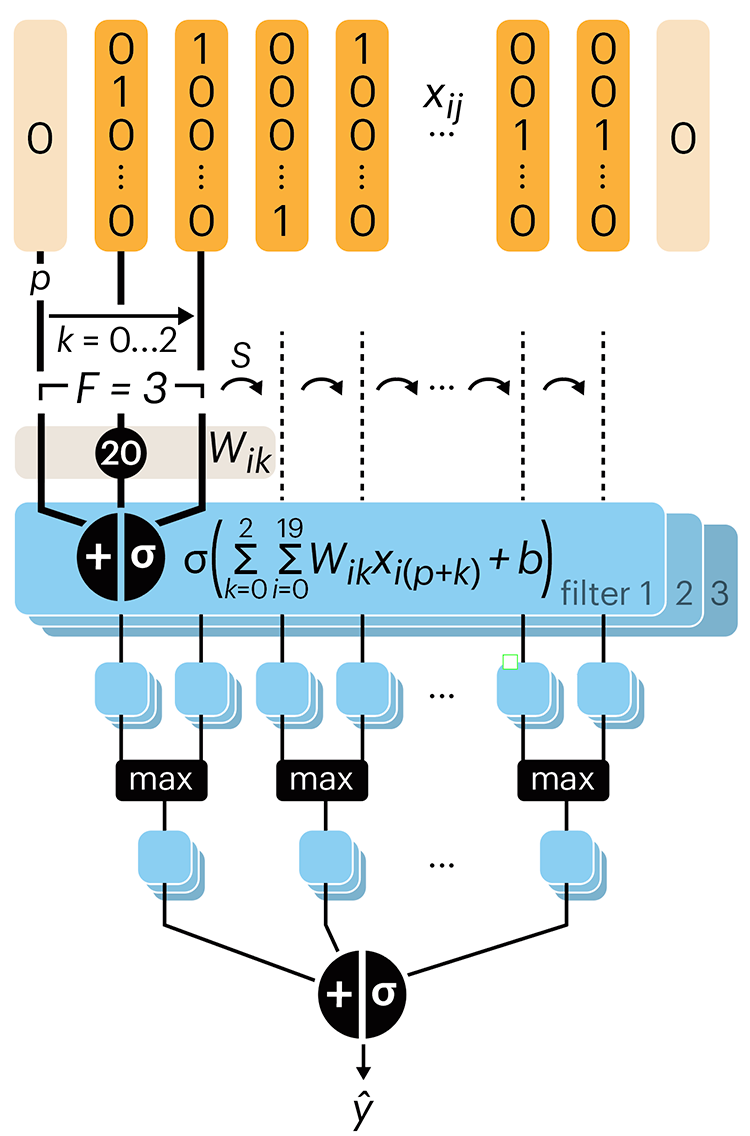
Even through convolutional networks have far fewer neurons that an FCN, they can perform substantially better for certain kinds of problems, such as sequence motif detection.
Derry, A., Krzywinski, M & Altman, N. (2023) Points of significance: Convolutional neural networks. Nature Methods 20:1269–1270.
Background reading
Derry, A., Krzywinski, M. & Altman, N. (2023) Points of significance: Neural network primer. Nature Methods 20:165–167.
Lever, J., Krzywinski, M. & Altman, N. (2016) Points of significance: Logistic regression. Nature Methods 13:541–542.